February's Hall of Famer

Hello all,
Please cast your votes for the Hall of Famer of the month of February. Cast your votes under comments, please. Thank you. The first place will receive 5 bonus points, second place 4, third 3, fourth 2, and fifth 1.
Good Luck.

Test #1

Hey Mr. P, I think I'll be alright with the test. (:

Test

Did the review , should be fine on it.

Hey mr.p do we have to know how to graph sin and everything like that? Or did you say that was onthe next test?

General Solutions of Trigonometric Equations

Today in class we learned about General Solutions of Trigonometric Equations.
What we learned was that General Solutions of Trigonometric Equations are:

• Not bounded by an interval
• Have unlimited coterminal answers
  

We also learned that

• For each solution of sinθ or cosθ in degrees, add 360°k or 2kπ for radians
• For each solution of tanθ in degrees, add 180°k or kπ for radians

For each answer, we include:

• Where kЄI (where k is an element of integers)
  

k accounts for all positive and negative coterminal integers

EXAMPLE of this type of equation




First step: Create your special triangle on unit circle

• Since tan is positive in quadrants 1 and 3, that's where we create our triangles

Second step: Figure out the angles for each quadrant they are in, making sure to add 360°or 180° or 2kπ or kπ


Q1 -> Ref. angle = Rel. angle
Q2 -> 180° - Ref. angle = Rel. angle
Q3 -> 180° + Ref. angle = Rel. angle
Q4 -> 360° - Ref. angle = Rel. angle

therefore
(in degrees)
Q1 = 30°+180°k, where kЄI
Q2 = 180°+30° = 210°+180°k, where kЄI

(in radians)
Q1 = π/6 + 2kπ
Q2 = 7π/6 + 2kπ


θ = π/6+2kπ , 7π/6+2kπ
where kЄI

Equation Question.

Step 1:


Step 2:


Step 3:


Step 4:


Step 5:


Step 6:


Step 7:


Step 8:


Step 9:

Solving Trigonometric Equations on a Specified Interval 2

In this topic, it is the extension of what we've discussed the other day. We solved more examples to challenge our skills on it. Like solving for the Θ over the indicated interval.

Taking down notes from Mr. Piatek:

For example : 2sin ² Θ - 3sinΘ - 1 over the interval 0 ≤ Θ ≤ 2π

Knowing how to factor:

2sin ² Θ - 3sinΘ - 1 (we can say that sinΘ=x)

*** in this equation, we can say it as simple as 2x ² - 3x + 1 = 0

To factor this : a = 2, b = -3, c = 1

then multiply: (a)(c) = (2)(1) = 2

*** Find two factors that of 2 that will add up to -3 (Find two numbers that when you multiply to 2 is positive and if you add will be negative)

Example: -2 and 1

= (-2)(1)

= 2

and -2 and -1

= (-2)(-1)

= -3

For the given example, 2sin ² Θ - 3sinΘ - 1 over the interval 0 ≤ Θ ≤ 2π

Solution:

- (2sin ² Θ)(sinΘ - 1)

- sinΘ = ½ sinΘ = 1

- sin 30° sin 90° = π/2

Find where sinΘ is positive,

Knowing now that it is in Q1 and Q2, draw a diagram having a reference angle of 30°,

As Q1 : Ref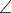 = Rel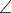

Θ = 30° / 30π/180° = π/6

As Q2 : π - Ref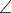

180° - 30° = 150° / 150π/180 = 10π/12 = 5π/6

Answer : Θ = π/6 , π/2 , 5π/6

NOTE: In using a calculator, be sure to set it to radians or degree if needed.

Hey Mr. P

I have a question with the worksheet; #6 & #18, I hope we could go over them in class. Thanks (:

Solving Trigonometric Equations on a Specified Interval

We started to study "Solving Trigonometric Equations on a Spcifid Interval" and it shows how to get θ in degrees or radians by using CAST rule.

FORMULA EVERY QUADRANT:
QI : Ref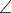 = Rel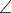
QII : 180 - Ref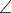 = Rel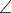
QIII: 180 + Ref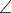 = Rel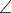
QIV: 360 - Ref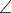 = Rel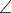

Example: 2cosθ + = 0 over the interval

• 2cosθ + = 0
  
• 2cosθ = - : Transpose and it should be -
• : After transpose, divide both sides by 2 and,
  

• : you will know now your cosθ

Then diagram it and the reference angle should be in QII and QIII because cosθ is (-) in QII and QII

QII: 180 - =
QIII: 180 + =

θ = {, }

Special Angles and the Trigonometric Functions 2

Special Angels and the Trigonometric fucntions 1

yesterday we have learn in class is about special angel and the trigonometric fucntions 1 to determine the exact value of trigonometric ratios for any multiples of 0°, 30°, 45°, 60° and 90° : as well as 0 , π/6 , π/4 , π/3 and π/2.

Six Trigonometric Functions

Sinθ = O/H Cscθ = H/O

Cosθ = A/H Secθ = H/A

Tanθ = O/A Cotθ = A/O

Special Angles - most angel on the unit circle are base on referenceangles of either 30°, 45° or 60°.

To find out solve the equation first. Select possible quadrant using CAST rule. Than check the interval given. By using the soh cah toa determine the reference angle by substituting the value of Sin θ = Opposite/Hypotenuse in a triangle in selected quadrant for this example. In last by using 3 simple steps we can find the related angle.

The Unit Circle (part 2)

Last Friday we learned about the unit circle. Our formula in unit circle is X squared+ Y squared= 1. The standard position starts at (1,0).
In the CAST rule :
Quadrant I : sin(theta)- positive
cos(theta)-positive
tan(theta)-positive
Quadrant II : sin(theta)-positive
cos(theta)-negative
tan(theta)-negative
Quadrant III : sin(theta)-negative
cos(theta)-negative
tan(theta)-positive
Quadrant IV : sin(theta)-negative
cos(theta)-positive
tan(theta)-negative

Examples: 2 (stands for squared)
Find the sin(theta) in Quadrant I:
Given: (square root of 5, 2)
a2=b2=c2
square root of 5 squared=c2
5+4+c2
c2+square root of 9
=3
sin(theta)=O/H
=2/3

If (5/13, y) is a point on the unit circle in quadrant IV, find the value of y.
y=-12/13

The point P on the unit is NOT in Quadrant I. If sin(theta)=8/17, find the value of cos (theta).
cos (theta)=A/H
=-15/17

Find the tan(theta) in Quadrant II.
Given:(-4, 18)
tan(theta)=O/H
=-18/4 simplify: divide it to two.
=-9/2

Find the cos(theta) in Quadrant II.
given: (-3, 4)
cos(theta)= A/H
2 (stands for squared)
=a2+b2=c2
= -3 squared+ 4 squared=c2
=c2 square root= square root of 25
=5

cos(theta)= A/H
=-3/5

Homework?

Hey Mr.P,

The assignment you said that was to be done over the weekend , is that found on the wiki?If not I'll just come and get it in the morning then finish it by class starts.

Thanks

The unit circle

The unit circle

In today's class we learned about unit circles. Unit circle is a circle with its centre at the origin and with a radius of one unit.

The equation for unit circle is: x squared+ y squared= one
A few class's ago we learned that positive distance is measured in a counter clock wise of a circle,and negative distance is measured in a clockwise of a circle.

we learned that the notation p(theta) is used to denote the terminal point,where the terminal arm of angle theta intercepts the unit circle, for every arc length theta on the unit circle, p(theta) is unique.
we can define p(theta) as the ordered pair p(x,y)

we were also reminded of SOH, CAH, TOA and what they stand for:

sin(theta)=opposite over hypotenuse

cos(theta)=adjacent over hypotenuse

tan(theta)=opposite over adjacent

using SOH ,CAH ,TOA we can found the p(theta) of an angle like we did in class,we found that:

sin(theta)=Y over 1 which sin(theta) equals Y

cos(theta)=X over 1 which cos(theta)equals X

tan(theta)=y over X

All that means is that the y axis is sin(theta) and x axis is cos(theta). Therefore, p(x,y)=p(cosO,sinO),and since the equation for unit circle is x squared+ y squared= one, it would be cos(squared) theta +sin(squared)theta=one.

And we were also reminded of the four quadrant and the rule of CAST.

quadrant 1 A quadrant 2 S quadrant 3 T quadrant 4 C


sin(theta) is + sin(theta) is + sin(theta) is _ sin(theta) is _

cos(theta) is + cos(theta) is _ cos(theta) is _ cos(theta) is +

tan(theta) is + tan(theta) is _ tan(theta) is + tan(theta) is _

Now one example of what we learned today is: where does p(theta) lie, given 0<2pi>

Degree and Radian Measure 2

Feb. 8th Monday

Today we learned how to convert degrees into radian and radian into degrees.
To convert degrees into radian you must multiply by pi/180.
To convert radian into degrees you must multiply by 180/pi.
Somtime when converting degrees into radian you will get #pi/#. If this happens you must reduce the two #'s as far as possilbe.
Sorry I didnt put any example...I'm feeble and i dont know how to on this :( and i couldnt find the pi sign so i just had to spell it lol

360 Degrees

There is 360 degrees in a circle because the sumerians invented it for writing back in 3000 BC. But back in 2400 they made a calender that divided 12 months into 30 days each. The sumerians saw the sun,moon and other planets moving around in that degree. That is where the origin of 360 came from!

why are there 360 degrees in a circle?

Add caption

An ancient group of people, known as the Sumerians, invented a calendar around 2400 BC that divided the year into 12 months with 30 days each, totaling 360 days. They noticed the circular motion of the Sun across the skyand they divided its circular path into 360 degrees that tracked each day's movement of the Sun.